Music and the art of relativity
The fact that there are tuning pegs (or tuning adjustments available) on virtually all musical instruments underlines the important Universal principle of relativity that is critical to the art of 'music'.
Just before an orchestra begins a concert, every single musician tunes his/her instrument to the same note (usually an 'A') played by the lead violinist. The resultant drone of notes rising and falling to meet in concord with this long-bowed sustained particular pitch or frequency holds a certain fascination in itself even though it lasts mere seconds— one quickly senses the resolving unison of timbres which enriches the leading first-call of the violin. And just as quickly as this unison is reached, silence replaces it, awaiting the conductor's entrance to the stage and podium... and the anticipation of the opening chords.
I am reminded of Saint-Saëns...
Over the years I have painted many studio pictures to music and especially to the Saint-Saëns Symphony No. 3 in C minor. From the Romantic Period of music history, it is a musical composition that typifies the era in being scored for a large orchestra (but here also includes a grand piano and pipe organ). It is a work of startling contrasts, imploring passages, urgent rhythms, sweeping vistas, majestic crescendos and the tumult and reverberant rumble of the deep pipes contrasted with lyric cascading melodies. It is a masterpiece of truly epic proportions enfolded and interwoven within the self-imposed restriction of just two main movements (breaking with the traditional four of the symphonic form refined during the Classical Period).
The octave and the ratio of a half
The two movements of Saint-Saëns' symphony echo another great musical insight stretching back to at least the time of the Pythagoreans: the relation between harmonious intervals and whole-number divisions of a vibrating string. The first and most irreducible of such divisions is the ratio of a half, or 1:2.
Occluding a vibrating violin string against a fingerboard at this precise half-way point will produce a note (when bowed or plucked) that sounds to the human ear and mind 'like the same note' except higher. This is an incredible neurophysiological fact of perception which I do not believe is merely culturally conditioned. It transcends our own time and space and is profoundly connected to a Universal resonance.
The interval between the base note and the latter one (produced by halving the length of the string) is called an octave. The name reflects the ubiquitous diatonic scale of the classical tonal system of music with its eight notes and whose mathematical proportions were later 'tweaked' and related or 're-tuned' (by JS Bach) to synchronise with twelve smaller and equally-spaced subdivisions of the octave, and these smaller intervals are today known as semitones. This great insight and invention allowed composers to modulate from one key to another, and JS Bach, 'the artist and scientist', empirically explored the possibilities of modulation opened up by the new scale structure by composing works in every key signature (based upon each semitone of the octave) within his now-famous set of compositions, the 22 Preludes and Fugues. [We observe that eleven semitones bring us to the twelfth, which concurs with the octave or 'tonic', and has the same key signature as the base note, hence Bach only needed to explore compositions based on the 11 semitones within the span of an octave of his well-tempered klavier. Upwards and downwards, this was 2 x 11, or 22.]
Musical scales and other whole-number divisions of a vibrating string
The diagrams reproduced below are from the accompanying book upon which this web site is based (W. Roberts, 2003, pp. 14-15). From left to right, it shows firstly the octave (based on halving the length of the string), then the next two most important intervals used in the western tonal system of musical scales—those of the fifth and fourth (known in music as the dominant and subdominant respectively). The interval of the fifth (or 'dominant') divides the string in the ratio of 2/3, and the interval of the fourth (or 'subdominant') divides the string in the ratio of 3/4.
It is indeed a remarkable mystery that the first four numbers play such a pivotal role in musical scales and in determining harmonious combinations (chords) which, while adding to the richness of the texture of sound, also retain the relativity among integers (whole numbers) as their basis. That the human mind also resonates to such scale structure intervals (and certain combinations of them) is perhaps not unexpected in light of the material presented herein, but remains an equally remarkable synchronicity.
 |
|
Further whole-number ratios created intervening intervals in a major or minor diatonic scale thus—
|
|
|
|
Some readers may more easily visualise why these whole-number intervals are so important if we re-draw the above diagram, but this time illustrate how such waves on a stringed instrument can perfectly fit the string a whole-number of times. Waves that 'fit a whole-number of times' are known in physics as 'standing waves', and it is not hard to imagine why they are called that, nor to imagine that such standing waves may well outlast the sound of non-standing waves which, by contrast, do not fit the string-length a whole-number of times and which tend to sound dull and to fade extremely rapidly—because the latter are 'non-resonant'.
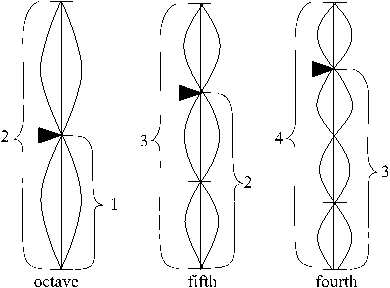
Figure reproduced from my book (W Roberts, 2003, p.15)
| back to top |
next > |