The area of any scalene triangle in etu
This web page is quoted from the author's book Principles of nature; towards a new visual language, WA Roberts P/L Canberra. 2003. [Used with permission of the author]
We are now ready to look at scalene triangles. These are triangles having all sides unequal.
We first recall a rather simple but beautiful property of triangles inscribed in parallelograms. This will simplify the analysis of scalene areas enormously.
Let 
ABC be any scalene triangle (fig.60).
Figure 60
Construct a 60° parallelogram around ABC as in figure 61(a).
Figure 61(a)
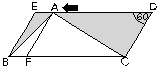
Figure 61(b)
Note that vertex A of ABC can ‘slide’ along ED without affecting the area of ABC since it retains the same base BC and the same altitude during these transformations.
From figure 61(a), we observe that the original scalene triangle has an area which is exactly half that of the parallelogram BEDC for the following reasons. The line AF (parallel to BE and CD), divides BEDC into two smaller parallelograms,
each of which contains a pair of congruent triangles. Thus the area of BAC (which equals half the area of parallelogram BEAF plus half the area of parallelogram FADC), equals half the area of the parallelogram BEDC.
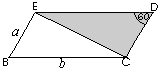
Figure 62
Notice how similar this is to the most well-known way (today) of finding the area of a triangle in square units, that is, as given by the formula 1/2bh, where h is the perpendicular height of the vertex from a defined base b. Expressed in etu’s, we have a similar but even simpler formula for the area of any triangle . We no longer have to halve the product of 'base x height', and the height has become the 60° altitude of the vertex instead of the perpendicular altitude (fig. 64).
Areascalene triangle = ab
where a is the 60° altitude of the vertex from base b and where the area is given in etu.
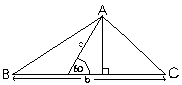
Figure 64 Area in square units = (1/2)bh |
| back
to top |
next > |