From counting to abstraction in math
How did the language of mathematics develop? And what was it that enabled us to use this language not only to manipulate quantities and shapes, but ultimately to model our world and Universe, to successfully predict eclipses and the return of comets? If the language of number was merely an expedient invention of ours to facilitate human affairs, why should this mathematical language be one which the Universe apparently not only understands, but itself speaks?
| Clues to the interconnectedness of number and nature | |
|---|---|
 |
Simple reckoning or counting first consisted in one-to-one parity matching. For example, one pebble for each of the sheep within a flock. Although apparently simple, this necessitated the application of the sophisticated and abstract concept of the unit. (See text below)
|
 |
The Pythagoreans found that certain numbers of units could be arranged geometrically. |
|
Pythagoreans discovered a consistent proportion among the sides of any right triangle. Thus there not only existed casual connections between number and geometry (such as arranging pebbles into geometric shapes), but also more rigorous connections that could be discovered. This led to the notion of proof, and to a formal syntax of number and shape we now call 'mathematics'. Depicted is the famous 3-4-5 right triangle, showing that, 32 + 42 = 52
|
 |
The Pythagoreans also discovered a connection between whole number ratios and intervals of sound that could be combined to form chords which appealed to the human hear. These harmonious combinations of notes, intriguingly coincided with the divisions of a vibrating string in whole-number ratios. For example, the ratio of 1:2 (i.e. halving the length of a vibrating string) resulted in a note that sounded the same as the original, except higher. Today, we call it an 'octave'. |
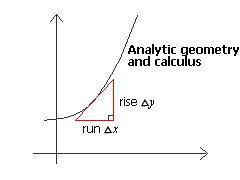 |
The right angle and right triangle assumed pivotal roles in co-ordinate and analytic geometry. This paved the way for algebraic descriptions of lines (equations), curves, and geometric shapes. This in turn, established another connection between number and geometry. |
 |
The right triangle and Pythagoras' theorem opened up the field of trigonometry. The proportions of the less regular triangles were able to be elucidated and mediated through the known and regular proportions of the right triangle as defined by the Pythagorean theorem. |
 |
The key mathematical tool used by Einstein in his theory of Special Relativity was the theorem of Pythagoras. |
 |
In his theory of General Relativity, Einstein goes on to describe gravitation in terms of the geometry of space-time. |
The story of number is a fascinating one of enormous scope and diversity. Even simple counting turns out to require strikingly abstract thinking. It is a much bigger jump than might first be imagined which takes us from the qualitative many to the quantitative reckoning of absolute number. For the human eye is generally not adept at perceiving in a glance quantities greater than four. We can recognise one, two, three, or four of most things, but beyond that, we have to to count the elements within a set in order to determine their exact number. So the ability to count required much more than a normally functioning visual system.
The first abstract idea?
Although the capacity to count to four seems rudimentary, there was something quite abstract —a beguilingly simple idea —which served as the essential element without which, even this, would have been an untenable nonsense. Namely, the idea of the unit or quantum, the recognition of the wholeness and discreteness of an entity. Even words lose their meaning unless there is subsumed within language a presupposition of the unit —nouns make no sense unless there exist units or discrete things which may be ‘named’.
The idea of the unit is perhaps as close as we can come to the bedrock of all mathematics and thinking to follow. So fundamental is the unit to arithmetic that the Greeks did not consider "1" to be a number at all but rather the monad or indivisible from which all other numbers arose. Abstraction and the seeds of algebra were born here: 'let this animal be one'; 'let this finger or this stone represent that animal'. The recognition of unitariness went beyond a recognition of similarities. Counting things which are different meant you had to abstractly assign unitary value to each object.
Counting things which are different meant you had to abstractly assign unitary value to each object. |
|
The abstract concept of the unit was a key that opened up thinking as we understand it today. It formed a principle of connection among things that, superficially at least, appeared to be unrelated.
Seeing the one within the many
The notion of assigning unitary value to each of the members of a heterogeneous set simultaneously spawned the notion of plurality or 'the many'. Before counting became common practice in human affairs the differences between discrete entities (be they sheep, people, trees, etc) were reflected within language by the multitude of words, names, and symbols applied to this diversity. But the introduction of counting saw a subtle attuning of human perception towards the similarities among the members of a set. That is because, in counting, every entity was considered to be the same: each was a discrete unit.
Whilst the concept of the many identified the condition of plurality, its weakness lay in its broad and qualitative vagueness. Another more pointed question therefore arose this time focusing on quantitative distinctions. That question was, How many? It was tackled by diverse, simple, and at times ingenious means in different cultures and periods, and from this diversity, certain underlying patterns of thinking and arrangements of numerals emerge as the germinal ideas that would lead on to a future language of number whose power and sophistication very few could have foreseen.
The abstraction of number begins to lose its bearings
—number notations begin to go awry
The history of mathematics saw a gradual abstraction in the way numbers were represented (symbolised). Although it was empowering, the emerging rhythms and connections between and among numbers (as abstract concepts) began to get a little out of step over time.
One problem was (and remains) the fact that the numerals continue to bear little or no correlation to the quantities they purport to represent. For example the numeral "8" does not resonate (as a sign) with the quality of an 'octave' nor eight as a numerical quantity. Neither does "7" bear any logical figurative connection to the quantity nor even the concept of the seventh position (an ordinal idea). These numerals we learn by rote. The fact that a mere ten signs (the numerals: 0, 1, 2 ,3, 4, 5, 6, 7, 8, 9, ) were required to be learned in order to represent and communicate any conceivable quantity was and is a virtue of the incumbent system, and has meant that, for thousands of years, we have accepted this small alphabet of numerals as 'a given', passing on (what is in other respects a certain kind of 'dissonance' within our current number notation) from generation to generation.
Such numerals used to this day are more suited, it seems, to a passing 'era of pens and paper'.
Future notations of mathematics will almost certainly highlight deficiencies within the current notation while, at the same time, hopefully lauding its strengths and paying homage to the pioneering work of an incredible lineage of great thinkers and innovators that have spanned time and the globe, acknowledging their positive contribution to mathematics (and thinking), and extending the language of number very far indeed.
| back to top |
next > |